DCE parameters

DCE Parameters

What quantitative parameters can be extracted from the DCE data?

Once the raw DCE signal data has been converted into gadolinium concentrations, the next step is to determine a tissue model to which the data will be fitted. One of the simplest and most widely used is that proposed by Paul Tofts and colleagues illustrated below.

Tofts Model. The relative sizes of the extracellular spaces are exaggerated compared the that of the non-gadolinium-containing intracellular spaces (dark blue). Moreover the fractional plasma volume (v_p, beige) is generally much smaller than the fractional tissue extravascular extracellular space (v_e, light blue).

Each voxel of tissue in the Tofts Model contains three components: tissue parenchymal cells (dark blue), blood vessels (containing erythrocytes and plasma), and the tissue extracellular extravascular space (EES). Gadolinium contrast, residing in plasma immediately after injection, is assumed to leak across the vascular endothelium into the EES by passive diffusion due to a concentration gradient. The influx mass transfer rate of gadolinium (K^trans) depends on blood flow, vascular surface area, and vascular permeability. A corresponding reflux rate of gadolinium from the EES back into plasma is denoted k_ep. Gadolinium contrast does not enter cells, so its concentration depends on dimensionless fractional volumes of plasma (v_p) and the EES (v_e) denoted in the diagram.

Two basic mathematical formulations of the Tofts model exist (available for the mathematically curious under the Advanced Discussion tab below). The first of these assumes that the plasma component (vp) is small and makes little contribution to the total tissue MR signal. The second (revised) formulation of the Tofts model includes this plasma contribution, and (although adding complexity) is probably more appropriate for representing highly vascular lesions such as malignant tumors. The meanings and practical use of the Tofts DCE parameters will be explored in the next several Q&A's.

Advanced Discussion (show/hide)»

Pharmacokinetic analysis using the Tofts Model

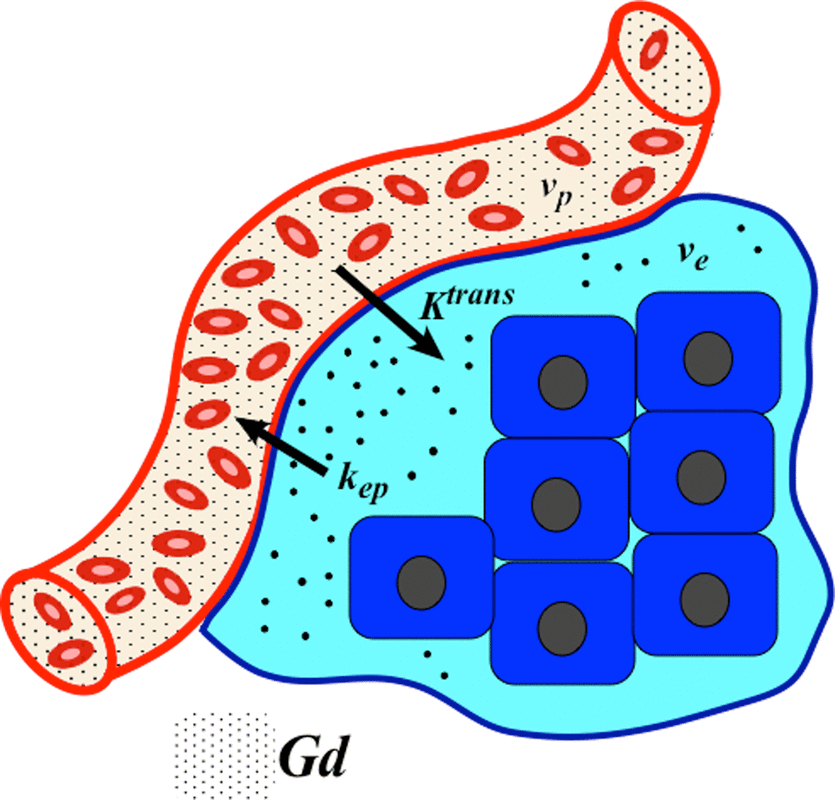
The original Tofts and Kermode (1991) model assumes that the equilibrium concentration of gadolinium in whole tissue, C_t, is driven by simple passive diffusion based on its concentration difference between plasma (C_p) and the tissue extravascular extracellular space (C_e). Since gadolinium contrast does not enter cells, C_e is higher than C_t by the factor (1/v_e), where v_e is the dimensionless volume fraction (0<v_e<1) of the tissue extravascular extracellular space (EES). The defining Tofts-Kermode equation is therefore

where K^trans is the mass transfer influx constant measured in units of min⁻¹. Note that the right side of the equation can be re-written as

with k_ep defined as K^trans/v_e, representing the (reverse) transfer rate for contrast from the EES back into plasma. Note that K^trans, v_e, and k_ep are interrelated and thus not all independent parameters. If two are known, the third can be computed.

In a DCE study we are able to determine the time-dependent gadolinium concentration in plasma, C_p, using either an arterial input function (AIF) or estimating it assuming bi-exponential decay from an initial value resulting from renal clearance and whole body extracellular space distribution. Likewise, by measuring the signal intensities of tissue we can determine the tissue gadolinium concentration, C_t. We do not know either K^trans or v_e, however. These are potentially important physiological parameters of interest that can be estimated by fitting the measured data to the Tofts model.

The original Tofts-Kermode model assumed the intravascular contribution to total tissue gadolinium concentration was negligible. In other words, the fractional volume parameter v_p is very small and disregarded in the analysis. The simple TK model thus has only a single compartment with two free parameters, whose differential equation can solved by integration to yield

Using nonlinear least squares estimation on a voxel-by-voxel basis, K^trans and v_e can then be computed.

The above formulation assumes that the intravascular contribution to total tissue gadolinium concentration is negligible and that C_t arises purely from gadolinium in the EES. Although this may be true for lesions such as multiple sclerosis plaques and low-grade tumors, it is certainly not the case for many malignant tumors and other highly vascular lesions.

A common modification of the original Tofts model takes this vascular contribution into account, introducing a new parameter v_p, the volume fraction of total tissue containing plasma. Like its counterpart v_e, v_p is dimensionless as it represents blood plasma volume per unit volume of tissue. This expanded Tofts model now has 2 compartments and 3 parameters, admitting to the following solution including a new term (v_pC_p)

It should be noted that adding more free parameters to a model does not necessarily improve it. More data points are needed to estimate the additional variables. Nonlinear least squares fitting algorithms, already somewhat unstable and ill-posed, may suffer from the inclusion of more noise and converge to unrealistic values.

Other Tissue Models for DCE Imaging

A pharmacokinetic model was developed by Brix and colleagues in Germany at the same time Tofts and Kermode were working in England. The original Brix formulation, now expanded, was an open-exchange model with central (vascular/plasma) and peripheral (tissue extracellular space) compartments. Forward and reverse gadolinium exchange rates between the compartments were denoted K₁₂ and K₂₁, respectively, corresponding roughly to K^trans and k_ep of the Tofts model. However, the Brix model explicitly separated flow from permeability effects (which are confounded in the K^trans concept). The original Brix model also used a rather inaccurate method of estimating gadolinium concentration from percent signal changes, but had the advantage that it did not require precontrast tissue T1 mapping nor measurement of an arterial input function.

Distributed parameter models, also known as tissue homogeneity models, abandon the concept of "well-mixed" compartments as used by Tofts and Brix. Here a gadolinium concentration gradient is assumed to exist along the length of each capillary. This requires incorporation of transit time into the model but allows the potential of extracting blood flow information directly from the analysis.

The Patlak model has recently become popular for the analysis of subtle areas of increased blood-brain-barrier permeability (which may be a clue to the pathophysiology of Alzheimer disease). Like the others, the Patlak model describes a highly perfused two-compartment model. The major difference is that contrast diffusion from the blood plasma to the extravascular extracellular space (EES) is considered to be unidirectional. The tracer concentration in tissue C_t(t) is given by

C_t(t) = v_pC_p(t) + K^Trans ∫C_p(τ) dτ

A linear graphical analysis of the ratio C_t(t)/C_p(t) regressed against ∫C_p(τ)dτ/C_p(t) produces a straight line with slope K^Trans and y-intercept v_p.

References
Brix G, Semmler W, Port R, et al. Pharmacokinetic parameters in CNS Gd-DTPA enhanced MR imaging. J Comput Assist Tomogr 1991; 15:621-628.
Heye AK, Culling RD, del C Valdés Hernández M, et al. Assessment of blood-brain barrier disruption using dynamic contrast-enhanced MRI. A systematic review. NeuroImage: Clinical 2014; 6:262-274. [DOI LINK]
Manning C, Stringer M, Dickie B, et al. Sources of systematic error in DCE-MRI estimation of low-level blood-brain barrier leakage. Magn Reson Med 2021; (in press) [DOI LINK] (Casts doubt on accuracy of Patlak model for measuring BBB disruption)
Tofts PS. Modeling tracer kinetics in dynamic Gd-DTPA MR imaging. J Magn Reson Imaging 1997; 7:91-101.
Tofts PS, Brix, G, Buckley DL, et al. Estimating kinetic parameters from dynamic contrast-enhanced T1-weighted MRI of a diffusable tracer: standardized quantities and symbols. J Magn Reson Imaging 1999; 10:223-232.
Tofts PS, Kermode AG. Measurement of the blood-brain barrier permeability and leakage space using dynamic MR imaging. 1. Fundamental concepts. Magn Reson Med 1991; 17:357-367
Zaharchuk G. Theoretical basis of hemodynamic MR imaging techniques to measure cerebral blood volume, cerebral blood flow, and permeability. AJNR Am J Neuroradiol 2007; 28:1850-8.

Related Questions
Is Ktrans the same as permeability?
How do calculated DCE parameters relate to patterns of enhancement we see on clinical images?

← Previous Question

Next Question →

↑ Complete List of Questions ↑