Net Magnetization (M)

What is net magnetization and how does it apply to NMR?  

Net magnetization (M), the averaged sum of many individual quantum spins, can be treated as a regular vector in classical physics.

Fortunately, nearly all the ideas behind MRI can be explained in terms of classical physics. Having an appreciation for quantum mechanics adds a certain perspective but is largely unnecessary.

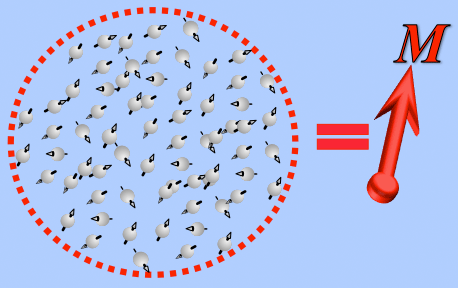
Although particle physicists must address the quantum world regularly, those involved with clinical or research MRI seldom do. The reason is straightforward —  During an MRI experiment we are not looking at the signal from individual nuclei, but from millions to billions in the aggregate. 

As shown in the diagram above, it is preferable not to think about individual nuclei (with their "freaky" quantum behavior).  Instead you should think about the sum of their magnetic properties averaged together to create a net magnetization (M). Net magnetization (M) behaves as a "regular" vector using the principles of classical physics.  From this point forth we will almost exclusively use the vector M rather than individual nuclear spins when explaining aspects of NMR and MRI.

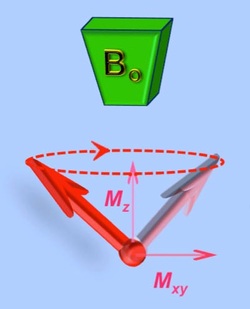
At equilibrium (i.e., when no scanning is taking place) M is aligned with the main magnetic field (Bo). However, during the MR imaging M is purposely tipped out of alignment allowing it to precess around the direction of Bo (just as the individual spins that compose M also precess around Bo). The direction of the Bo field is commonly designated as the z-axis.  Using this coordinate system, M can be considered to have both longitudinal (Mz) and transverse (Mxy) components.  Since M is precessing, in general Mz and Mxy will be a function of time.

The net magnetization (M) is a vector that can be resolved into components longitudinal (Mz) and transverse (Mxy) with respect to the main magnetic field (Bo). At rest (between imaging sequences) M is aligned with Bo and hence Mz = Mo us a maximum and Mxy=0.

Advanced Discussion (show/hide)»

In more formal analysis, the net magnetization vector (M) now operating under the laws of classical physics is considered the expectation taken over the entire ensemble. When the results of an experiment depend only upon the statistical behavior of a large number of quantized particles, an explanation in terms of classical (non-quantum) physics is generally possible. This is indeed the case with NMR, in which the expectation value of the magnetization may be shown to have any orientation to the magnetic field, ranging from parallel to antiparallel and including all values between.

---

References
      Hanson L. Is quantum mechanics necessary for understanding magnetic resonance?  Concepts Mag Reson Part A 2008;32A(5):329-340.

---

Related Questions
     Nearly all the books and papers I have seen show diagrams of protons flipping from spin-up to spin-down states. Are you telling me these are all wrong?  
     What is spin?

---

↑ Complete List of Questions ↑

←  Previous Question

Next Question  →