Methods for filling k-space

You alluded to different trajectories for filling k-space. What are they?  

k-space for pigeons

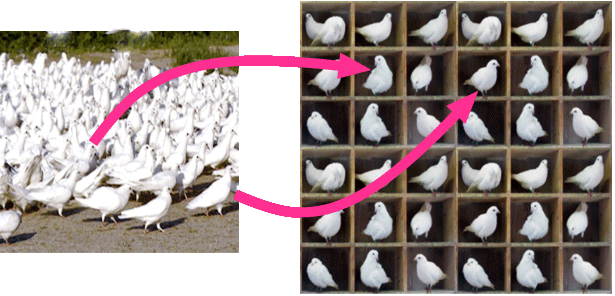
Before generation of the MR signal, k-space is just an array of blank cells awaiting the arrival of data. As an analogy, think of it as a box of empty "pigeon holes" waiting to receive "pigeons". The goal is to put one pigeon in each hole. As long as the entire box gets filled, the order is unimportant.

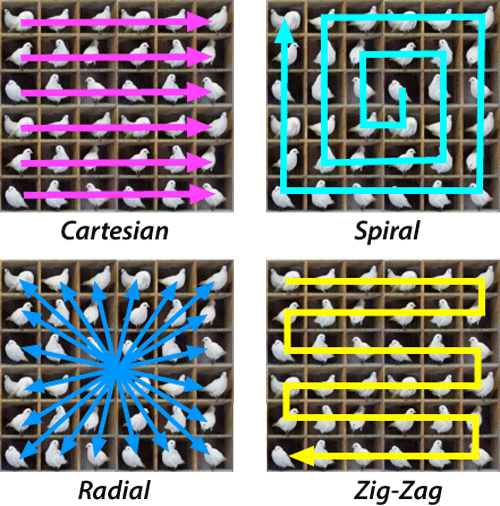
To the right are four common k-space trajectories used in modern MR imaging. Traditionally the Cartesian (row-by-row) method was used nearly exclusively, but today all these patterns are widely encountered. 

Standard sequential Cartesian filling of k-space with left-to-right frequency-encoding. (Courtesy of Brian Hargreaves)

Centric ordering of k-space acquisition. (Courtesy of Brian Hargreaves)

Outward radial ordering of k-space. (Courtesy of Brian Hargreaves)

Advanced Discussion (show/hide)»

Choosing among the various k-space traversal strategies depends on the application. The choice is perhaps most critical for echo-planar imaging (EPI), where the long readout train makes the sequence highly vulnerable to susceptibility artifacts and rapid switching produces unwanted eddy current effects. The usual analytic approach for understanding these effects decomposes them along the three standard imaging axes (phase-encoding, frequency-encoding, and slice-select).

Gradients along the frequency-encode direction cause image shearing and blurring that are proportional to the length of the readout train. Gradients along the phase-encode direction will rescale the image and change the effective TE. Because the bandwidth along the phase-encode axis is typically much lower than the other directions, off-resonance spins (such as fat protons) will be noticeably displaced. Thus EPI sequences typically employ fat saturation to reduce chemical shift artifacts in the phase-encode direction. Finally, gradients along the slice-select axis will dephase the signal and produce signal voids.

Cartesian patterns of traversing k-space are the easiest to implement and have long been the standard method for most MR imaging sequences, EPI or otherwise. Today, radial and spiral readouts are becoming the norm because they offer lower intrinsic sensitivity to motion and permit shorter TE values. The spirals may be "spiral in", "spiral out", or combined. Since there are not separate frequency-and phase-encode directions in radial and spiral techniques, the artifacts are different (they include curvilinear bands and ring-shaped blurring). Spiral scans are also more affected by incorrect gradient timing and concomitant field gradients. Image reconstruction is also more complicated, and may require "gridding" or other methods to warp the measured k-space points into a rectangular matrix for fast Fourier transform numerical processing.

---

References
     Mezrich R. A perspective on k-space. Radiology 1995; 195: 297-315. [review].
     Miller K. MRI image formation (ppt). On-line lecture notes available at users.fmrib.ox.ac.uk/~karla/teaching/image_formation.ppt

---

Related Questions
     Where do you get the data to fill k-space? How does this relate to MR signals and echoes?  

---

←  Previous Question

Next Question  →

↑ Complete List of Questions ↑